Without mathematics, there’s nothing you can do. Everything around you is mathematics. Everything around you is numbers.
What is Mastery of Mathematics?
Mastery of mathematics is not a fixed state but a continuum. At each stage of learning, pupils should acquire and demonstrate sufficient grasp of the mathematics relevant to their year group, so that their learning is sustainable over time and can be built upon in subsequent years.
This requires development of depth through looking at concepts in detail using a variety of representations and contexts and committing key facts, such as number bonds and times tables, to memory.
(Teaching for Mastery, NCETM 2015)
Our curriculum aims to embed the principles of a teaching for mastery approach underpinned with the belief that ‘every child can achieve in maths’.
Key Research
Research informed curriculum and pedagogical choices:
- https://content.ncetm.org.uk/mastery/NCETM_Primary_Teachingformastery_Report_July2019.pdf report carried out by the NCETM to record progress made by Teaching for Mastery schools in England.
- https://www.ncetm.org.uk/files/37086535/The+Essence+of+Maths+Teaching+for+Mastery+june+2016.pdf
The Essence of Maths Teaching for Mastery NCETM
- Mathematics Mastery
- Jerome Bruner (1915 – 2016) Cognitive development research.
- Richard Skemp (1919-1995) Relational Understanding and Instrumental Understanding. 1976
- Zoltan Dienes (1916-2014) Systematic Variation
- EEF: Mastery Learning + 5 months
Mastery learning | EEF (educationendowmentfoundation.org.uk) - EEF Guidance Improving Mathematics (2020):
Improving Mathematics in the Early Years and Key Stage 1 | EEF (educationendowmentfoundation.org.uk)
Improving Mathematics in Key Stages 2 and 3 | EEF (educationendowmentfoundation.org.uk)
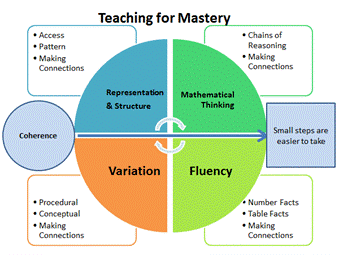
The 5 Big Ideas of Teaching for Mastery
Our curriculum and pedagogical choices are underpinned by the five big ideas of teaching for mastery:
(Taken from NCETM March 2017)
- Coherence
Lessons are broken down into small connected steps that gradually unfold the concept, providing access for all children and leading to a generalisation of the concept and the ability to apply the concept to a range of contexts. - Representation and Structure
Representations used in lessons expose the mathematical structure being taught, the aim being that students can do the maths without recourse to the representation - Mathematical Thinking
If taught ideas are to be understood deeply, they must not merely be passively received but must be worked on by the student: thought about, reasoned with and discussed with others - Fluency
Quick and efficient recall of facts and procedures and the flexibility to move between different contexts and representations of mathematics - Variation
Variation is twofold. It is firstly about how the teacher represents the concept being taught, often in more than one way, to draw attention to critical aspects, and to develop deep and holistic understanding. It is also about the sequencing of the episodes, activities and exercises used within a lesson and follow up practice, paying attention to what is kept the same and what changes, to connect the mathematics and draw attention to mathematical relationships and structure.
Mastery of Mathematics at Lansbury Lawrence
At Lansbury Lawrence, our maths curriculum has been designed to coherently sequence learning across EYFS to KS2. In response to the Pandemic, our curriculum was adapted and prioritised to meet the needs of all children in our school, which was supported through our partnerships with the NCETM, London North & South East Maths Hub and White Rose Maths.
Curriculum Aims:
Through our curriculum, we aim to develop mathematicians who:
- have a strong number sense
- can see patterns and make generalisations
- are articulate in their books and out loud
- make reasoned decisions and justify them
- think critically about their own and other’s strategies
- are equipped to solve unfamiliar problems in a range of contexts.
To achieve this our curriculum has:
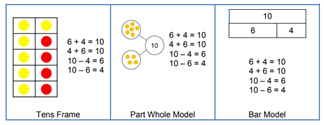
- a consistent set of concrete and pictorial representations from EYFS to Year 6
(NCETM 2015)
- ensures all children experience coherent and progressive models throughout the school to support deep and sustained learning.
- ensures lessons plan opportunities for children to make connections. For example:
- Additive reasoning
- Multiplicative reasoning
- Fractions with ratio
- Links to prior learning
- takes a problem-solving approach to modelled, guided, and independent work
- provides daily opportunities for children to meaningfully rehearse their number facts to develop fluency and confidence:
- Number Sense Maths Programme EYFS & KS1
- Multiplication Table facts in LKS2
- Arithmetic and Recapping KS2
- ensures that explicitly explaining and justifying process is critical when working as a class, in a group or independently.
- provides challenge and scaffolds so that all children move through the curriculum broadly at the same pace
- focuses on conceptual understanding
- Provides a framework of vocabulary, key terms and phrases.
For this to happen, our teachers are supported to:
- understand the fundamentals of number
- understand the progression from concrete to visual to abstract is not linear and each stage should be revisited as needed.
- use modelled and shared sessions to promote choice, critical thinking and a diversity of ideas.
- use a range of questioning that support all children to reflect on, explain and extend their thinking.
- scaffold work so all children reach the same goals
- explicitly model new learning using strategies such as the ‘silent teacher’ and worked example approach
- anticipate and teach away from misconceptions
- know potential sticking points in lessons and plan accordingly
- be precise in their use of vocabulary and expectation of children’s vocabulary
- know progression within each unit to assess, intervene and know when all children are ready to move on.
Our classrooms:
- map the progression of models used throughout the year
- have updated working walls that support the unit being taught
- are fully equipped with a range of manipulatives accessible to all the children all of the time.
Response to the Pandemic:
We followed advice from the DfE Teaching Mathematics in Primary Schools guidance; Teaching mathematics in primary schools – GOV.UK (www.gov.uk) which offered support by:
- identifying the core concepts that are needed for pupils to progress in their study of mathematics
- demonstrating how pupils can build their understanding of these concepts from year 1 to year 6
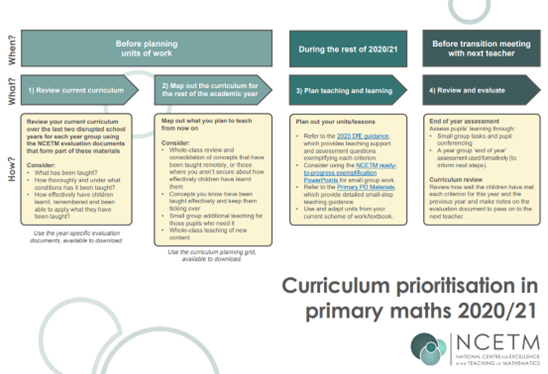
This supported our decisions on what to prioritise to ensure children developed firm foundations before moving on to new content. Our planning and review process is exemplified in the flow chart below:
One notable gap in children’s knowledge upon returning to school was number facts and quick mental recall of these to perform formal algorithms. To address this, we have timetabled 15-minute daily fluency sessions in all year groups:
- EYFS – KS1 Number Sense Maths (Systematic number fact teaching | Number Sense Maths)
- LKS2: Number Sense Maths and Multiplication Tables
- UKS2: Multiplication Tables and Arithmetic
These are regularly assessed and reviewed to support progress of all children.
NCETM Curriculum Prioritisation in Primary Maths:
From September 2021, our curriculum map was supported by the NCETM prioritisation framework which:
‘… provides coherent sequencing for the primary maths curriculum. It draws together the DfE guidance on curriculum prioritisation, with the high quality professional development and classroom resources provided by the NCETM Primary Mastery PD materials.’
We supplement this with White Rose Maths and online tools such as Learning by Questions.
End of Spring Term 1: Toys: Why have Toys changed over time?
As we reach the end of Spring Term 1, let's look back at all the exciting things we've learned this term! We’ve learned about toys from the past, practiced maths with doubles and place value, and written stories about lost toys. Let’s see what we've discovered! In...
CHRIST’S HOSPITAL YEAR 5 LONDON MATHS CHALLENGE
Monday 10th February, four wonderful mathematicians from Year 5 attended a Maths Problem solving competition at Guildhall. Representing East London and the only school from Tower Hamlets in attendance, our fearlessly focussed problem solvers took on five different...
Designing and Making a Bag for DT
For our DT project this term, year 5 are designing and making an eco friendly bag, in line with their learning theme which is Eco Warriors. They’ve been learning about some of the negative impacts that humans have on the environment, and some of the actions we can...
Year 6 Maths Breakfast Club
Children from year 6 have been arriving in school early to enjoy their breakfast and continue practicing their maths problem solving and reasoning skills. Muhsina who is attending states; 'It is fun, you get to do more maths and if you don't understand a concept there...
Year 6: Place Value within 1
This morning, Peggy Angus class worked collaboratively to explore place value within 1 using counters and place value charts. Using a ten frame, children also made connections 10 tenths make 1 whole and used this to identify how many hundredths make 1 tenth. Alexia:...
Exploring 3D Shapes Through Song and Fun
This week, our Year 1 students wowed us with their energetic and educational 3D Shapes Assembly. The event was filled with singing, laughter, and interactive learning as the children showcased their understanding of 3D shapes. Ezan was super excited to sin and share...
Year 2’s Fun Problem Solving Lesson: Math, Strategy, and Dice
On Friday, in Year 2, our children had a fantastic time working on problem-solving skills through a fun and interactive dice game! The challenge was all about addition, strategy, and a little bit of friendly competition. It was wonderful to see the children engaged...
Maths Leaders 2024-2025
Lansbury Lawrence are proud to announce our new Maths Leaders for this academic year. Children needed to apply for their positions and we thrilled to be selected to represent maths and develop their pupil leadership skills. Look out for a range of events children will...
Year 5 Wonder Maths Club
Children in year 5 have been enjoying maths problem solving challenges during lunch time maths club. In preparation for the Wonder Maths challenge (click the link for information Home) , children are engaging with pattern spotting, code breaking, 24 Game and making...
Patterns: Hamilton class learn about patterns.
Why do we learn about pattern? Children love to find patterns in the world around them. Patterns help children understand change and that things happen over time. Patterns are things that repeat in a logical way, like vertical stripes on a sweater. They can be...